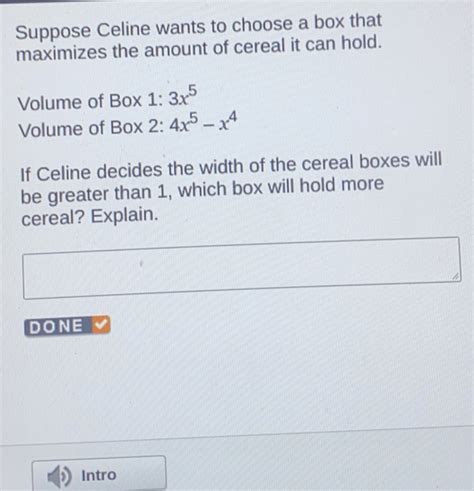
can hold. volume of box 1: 3x5 volume of box 2: 4x5 – x4 if celine decides The volume of Box 1 is (3*2^{5} = 96) and the volume of Box 2 is (4*2^{5}-2^{4} = 112).', 'From this, we can see that when x = 2, the volume of Box 2 is greater than the volume of Box 1. .
Rolex watches Datejust 36 1601. Rolex Datejust 36 1968 Vintage Rolex Datejust Ref. 1601-3 Engine Turned Bezel in Stainless Steel ( 14438) Year of production 1968 | No original box | No original papers. $4,900. Free insured shipping within: United States of America. Buy in monthly payments with Affirm on orders over $50. Learn more. Wire .
0 · suppose céline wants to choose a box that maximizes the
1 · suppose celine wants to choose a box that maximizes the
2 · suppose celine wants to choose a box that maximizes
3 · Suppose Celine wants to choose a box that maximizes the
4 · Solved: Suppose Celine wants to choose a box that maximizes
5 · Solved: Suppose Celine wants to choose a box that Which did
6 · SOLVED: Suppose Celine wants to choose a box that maximizes
$3,250.00
suppose céline wants to choose a box that maximizes the
If a box has a greater volume, it can hold more cereal. Volume of Box 1: 3x^5 Volume of Box 2: 4x^5-x^4 Box 2 can hold more cereal. Although the value of x is unknown, it represents a .
Volume of Box 2: 4x5-x4 Box 2 can hold more cereal. Although the value of x is unknown, it represents a fength, so it must be greater than zero. For any x>1, If Celine decides the width .We are given the volume formulas for two different boxes. Box 1 has a volume of 3x^5, and Box 2 has a volume of 4x^5 - x^4. We are also told that the width of the cereal boxes will be greater than 1.Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will .
fake bags online uk
suppose celine wants to choose a box that maximizes the
Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x^6 Volume of Box 2: 4x^6-x^4 If Celine decides the width of the cereal boxes will .
The volume of Box 1 is (3*2^{5} = 96) and the volume of Box 2 is (4*2^{5}-2^{4} = 112).', 'From this, we can see that when x = 2, the volume of Box 2 is greater than the volume of Box 1. .Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 _ x4 If Celine decides the width of the cereal boxes will .Volume of Box 1: 3x^(5) Volume of Box 2: 4x^(5)-x^(4) If Celine decides the width of the cereal boxes will be greater than 1 , which box will hold more cereal? Explain. Show MoreExplanation: To determine which cereal box holds more, we need to compare the volumes of the two boxes. For Box 1, the volume is 3 x 5, which simplifies to 15, considering the width x is .
For different values of greater than 1, the volume of Box 2 is consistently greater than the volume of Box 1. For example, let's check a few values: - For : Here, . - For : Again, . 4. Conclusion: - For , the volume of Box 2 ( ) is always greater than the volume of Box 1 ( ). - Therefore, if Celine wants to choose the box that maximizes the .
- Volume of Box 1: - Volume of Box 2: We are asked to find which box holds more cereal when the width of the cereal boxes is greater than 1, so let's consider a value , which is greater than 1, to make our comparison. 1. Calculate the volume of Box 1 when : 2. Calculate the volume of Box 2 when : 3. Now that we have the volumes for : - Box 1 .Which did you include in your response? Option 1: If a box has a greater volume, it can hold more cereal. Option 2: Box 2 can hold more cereal. Option 3: Although the value of x is unknown, it represents a length, so it must be greater than zero. Option 4: For any x > 1, the volume of box 2 is greater than the volume of box 1.First, let's substitute (since it's a value greater than 1) into the volume expressions and see which volume comes out larger. For Box 1, the volume is: For Box 2, the volume is: Now we compare the volumes: - Volume of Box 1: 96 - Volume of Box 2: 112 Since 112 (volume of Box 2) is greater than 96 (volume of Box 1), Box 2 can hold more cereal .
Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain.- For Box 2: 4. Compare the Volumes: - Volume of Box 1 = 96 - Volume of Box 2 = 112 Since 112 is greater than 96, Box 2 can hold more cereal than Box 1 for this given width greater than 1. 5. Conclusion: Therefore, if Celine wants to maximize the amount of cereal her box can hold and the width is greater than 1, Box 2 will be the better choice .Since the difference is positive for , it means that the volume of Box 2 is greater than the volume of Box 1 for these values of . Therefore, for , Box 2 will hold more cereal than Box 1. Celine should choose Box 2 if she wants to maximize the amount of cereal the box can hold when the width is greater than 1.
fake blood sealed in bag
Which did you include in your response? Option 1: If a box has a greater volume, it can hold more cereal. Option 2: Box 2 can hold more cereal. Option 3: Although the value of x is unknown, it represents a length, so it must be greater than zero. Option 4: For any x > 1, the volume of box 2 is greater than the volume of box 1.
- Volume of Box 2: ### For : - Volume of Box 1: - Volume of Box 2: Now let's compare the volumes for each width : - For : Box 1 holds 96, Box 2 holds 112 - For : Box 1 holds 729, Box 2 holds 891 - For : Box 1 holds 3072, Box 2 holds 3840 - For : Box 1 holds 9375, Box 2 holds 11875 In each case, Box 2 holds more cereal than Box 1. Thus, Celine .
- Volume of Box 2: - Substitute into the volume formula for Box 2: 4. Compare the Volumes: After evaluating the above calculations: - Volume of Box 1 is approximately . - Volume of Box 2 is approximately . Since the volume of Box 2 is greater than the volume of Box 1 (), Box 2 can hold more cereal when the width is greater than 1. Therefore .
To determine which box holds more cereal, we need to compare the volumes of the two boxes by considering a value of greater than 1, since the width of the boxes needs to be greater than 1. 1. Volume of Box 1: The volume is given by the expression . 2. Volume of Box 2: The volume is given by the expression . Let's evaluate these volumes using a value slightly greater than 1 for .Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. The length and width of box 1 is 3 and 5, respectively, and the length and width of box 2 is 4 and 5, respectively. If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain. Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain.
- A positive value for means that Volume of Box 2 is greater than Volume of Box 1. - Thus, for , Box 2 will hold more cereal than Box 1. So, if Celine wants to choose a box that maximizes the amount of cereal it can hold when the width is greater than 1, she should choose Box 2.So, there is no value of greater than 1 that satisfies . 6. Conclusion: Since cannot be less than 1 for volumes to work as required under , we conclude that under the condition : - Volume of Box 1 will always be less than Volume of Box 2 (). Therefore, Celine should choose Box 2 as it will hold more cereal when the width of the cereal boxes is .
Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain. 00:52. Packaging. The amount of cardboard needed to make the cereal box shown below can be found .Given the equation for the box's dimension as (4x-1)(x)(x^3), the degrees for length, width, and height would be 1,1, and 3 respectively. Length's degree: the term in the length with the highest degree is (4x-1) which is a degree of 1. Width's degree: The width is . Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain.
Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain. 01:40. FOOD Most cereals are packaged in cardboard boxes. If a box of cereal is 14 inches high, 6 . Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain.Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x5 Volume of Box 2: 4x5 – x4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain. 01:41. A company sells cereal in 2 different-sized boxes. DIMENSIONS FOR SMALLER CEREAL BOX .Suppose Celine wants to choose a box that maximizes the amount of cereal it can hold. Volume of Box 1: 3x^6 Volume of Box 2: 4x^6-x^4 If Celine decides the width of the cereal boxes will be greater than 1, which box will hold more cereal? Explain. Intro
Option B is correct: "Box 2 can hold more cereal. Although the value of x is unknown, it represents a length, so it must be greater than 0. For any x > 1, the volume of Box 2 is greater than the volume of Box 1." To determine which box can hold more cereal, let's compare the volumes of Box 1 and Box 2 step by step: Volume of Box 1: Volume of Box 2:Volume of Box 1: 3x^5 Volume of Box 2: 4x^5-x^4 Box 2 can hold more cereal. Although the value of x is unknown, it represents a length, If Celine decides the width of the cereal boxes will so it must be greater than zero. For any x> be greater than 1, which box will hold more 1, the volume of Box 2 is greater than the cereal? Explain. volume of .
Which expression represents the volume of this can? d. Use FOIL to explain how to find the product of(a + b)(a − b). . Celine's Cereal Company is launching a new brand of cereal and she is considering two different sizes for the base of the boxes. . Box 2: The length is 1 less than 4 times the width. The dimensions of the base of Box 1 .
- The coefficient of in Box 1 is 3. - The coefficient of in Box 2 is 4. Since 4 is greater than 3, the polynomial for Box 2 will grow faster than that for Box 1 as increases when . 4. Conclusion: - Therefore, for any value of greater than 1, Box 2 will hold more cereal than Box 1. Thus, Celine should choose Box 2 to maximize the amount of .
suppose celine wants to choose a box that maximizes

相片:Rolex雜誌. The first references that Rolex made available were the 6510 and 6511, many of which have 1955 serial numbers. Rolex filed the patent for the .
can hold. volume of box 1: 3x5 volume of box 2: 4x5 – x4 if celine decides|Solved: Suppose Celine wants to choose a box that maximizes